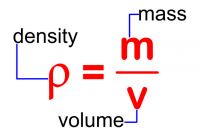Density Lab
| Density Lab | |||||||
|---|---|---|---|---|---|---|---|
| Type | Physics | ||||||
| Category | Lab | ||||||
| Latest Appearance | 2021 | ||||||
| Forum Threads | |||||||
| |||||||
| Question Marathon Threads | |||||||
| |||||||
| Official Resources | |||||||
| Website | www | ||||||
| Division B Results | |||||||
| |||||||
Density Lab, also known by the names Buoyancy Lab and Buoy Oh Buoy, is an event for the 2021 season. It previously was held in the 2019 season, the first season it was an official event, and the 2020 season. It was previously a trial event at the 2018 National Tournament. This event consists of two parts - a written test on density, buoyancy, concentrations, and the behavior of gases, and one or more hands-on tasks relating to those concepts.
Test Description
A Density Lab test consists of two parts, a written test, and at least one hands-on task. The written test is 50% to 75% of the total event score, while the hands-on portion contributes the remaining 25% to 50%. The event requires a basic knowledge of chemistry and physics as they pertain to density, concentrations, gas behavior, and buoyancy.
Students must use metric units with correct significant figures unless instructed otherwise.
Possible Hands-On Tasks
Students may be required to:
- measure or calculate the mass density of a given solid
- collect a volume of gas and calculate the volume, mass, and mass density
- determine the number density of multiple objects, such as the number of brown M&M's in a bag of M&M's
- determine the mass that a given helium balloon can lift
- determine the depth to which an object may sink in water
- determine the density of a material at different temperatures
- determine the buoyant force on an object submerged in a liquid
- build an aluminum foil boat that can hold as many pennies as possible using the materials provided
Most hands-on tasks will require students to make measurements using provided instruments. For this reason, it is recommended that students practice hands-on tasks using a variety of instrumentation and techniques.
Density
In general, density is a description of the amount of a substance per unit volume or area. There are several common expressions of density, including mass density, number density, and area density. Usually, the term density is used to refer to mass density.
Densities are expressed in a variety of different units, depending on what type of density it is and the units used to express the mass, number, area, and volume of the substance. All densities, however, are rates and thus their units follow the general form [Unit of mass or number] per [Unit of volume or area]. For example, the mass density of gold is approximately 19.3 grams per cubic centimeter.
In general, the density of a material is greatest when it is a solid and decreases as it changes phase to a liquid and from a liquid to a gas. This is not always the case, however; water is less dense as a solid than liquid. Likewise, within a single phase, density usually decreases with increasing temperature, a phenomenon known as thermal expansion. Conversely, substances within a single-phase usually increase in density with decreasing temperature, known as thermal contraction.
Mass Density
Mass density, usually just called density, is the mass of a substance per unit volume. It is usually represented by the lowercase Greek letter rho ([math]\displaystyle{ \rho }[/math]).
The formula for calculating density is shown in the image to the right.
Number Density
Number density is the number of countable objects per unit volume. It is given by the formula [math]\displaystyle{ \rho_{N} = \frac{N}{V} }[/math] where [math]\displaystyle{ \rho_{N} }[/math] is the number density of the objects, [math]\displaystyle{ N }[/math] is the number of the objects, and [math]\displaystyle{ V }[/math] is the volume of space being examined.
Number density can be calculated for any objects, though it is often used for the density of individual atoms or molecules. It is important to specify the object the number density refers to when writing the unit.
Area Density
Area density is the mass of an object per unit of surface area. It is commonly used for expressing density of objects such as paper or fabrics, whose thickness is not easily found using typical measuring instruments.
The formula for area density is [math]\displaystyle{ \rho_A = \frac{m}{A} }[/math] where [math]\displaystyle{ \rho_{A} }[/math] is the area density, [math]\displaystyle{ m }[/math] is the mass of the object, and [math]\displaystyle{ A }[/math] is the surface area over which the density is expressed.
Pressure
The pressure of any substance is defined as the force it exerts on another substance per unit area, perpendicular to this acted force. In terms of gases, it can also be defined as a measure of the number of collisions that gas molecules have with their container. An instrument used to measure pressure, typically in the atmosphere, is a barometer. The general formula for the pressure exerted on a substance is [math]\displaystyle{ P = \frac{F}{A} }[/math].
While its main application in this event is the gas laws, it may be useful to know the gauge pressure in multiple applications, such as a container full of said fluid on any object in the container or the bottom of the container itself. The solution to the calculation of this gauge pressure would be the product of the density, the gravitational constant, and the height of the fluid that is exerting this pressure on an object. The formula for this pressure is best known as [math]\displaystyle{ P = \rho gh }[/math]. Be careful with this formula though, because it can be a bit misleading in certain scenarios. For instance, if there is a pressure at the top of the fluid, such as atmospheric pressure, you must subtract the pressure on the top of the fluid from the pressure the fluid exerts on an object or surface below it. Also, make sure to keep in mind that hydrostatic pressure assumes that the fluid in this said container is water, which has a density of approximately 1 g/mL, as you may already know.
Another instrument besides the barometer that measures pressure is a manometer, a system where a gas tank is connected to a U-shaped tube filled with mercury. Depending on the side of the tube the majority of the mercury is on, the formula for the pressure of the gas may change. Though it is less likely to appear on a test, it is still good to know just in case. An image is shown below that shows the formula, dependent on the location of the mercury and how high or low it is on either side.
Concentrations
A concentration is an expression of the amount of one substance that is present in a given amount of a mixture of substances. Similar to density, concentrations may be expressed in multiple ways, including mass per unit mass ("mass/mass"), mass per unit volume ("mass/volume"), and volume per unit volume ("volume/volume").
Similar to density, the units used to express concentration will depend on the units of mass or volume used to measure the substance and mixture, but are rates and follow the general form [Unit of mass or volume] per [Unit of mass or volume]. For example, the concentration of salts in seawater is approximately 3.5 grams per liter.
When a concentration is mass/mass or volume/volume and the mass or volume of the substance and mixture are measured in the same units, the concentration may also be expressed as a proportion in percent, parts-per-million, or parts-per-billion notation. For example, if one gram of red dye was added to 100 grams of water, the concentration may be expressed as 0.01 grams (of dye) per gram (of water), or 1%. If expressing a concentration as a proportion, it is necessary to specify whether the proportion refers to a mass/mass or volume/volume concentration by stating "by mass" or "by volume" afterward. In the previous example, this would be done by stating the concentration as "1% by mass".
Gas Laws
Gas laws describe the relationship between key variables of gases. These variables include:
- Temperature (T) - a measure of the average kinetic energy of gas molecules; usually given in units of kelvin (K)
- Pressure (P) - a measure of force per unit area resulting from collisions of gas molecules; usually given in units of pascals (Pa), equivalent to one newton per square meter
- Volume (V) - the amount of space occupied by the gas; usually given in units of cubic meters (m³)
- Amount of substance (n) - the number of atoms or molecules of the gas; usually given in units of moles (mol), equivalent to 6.022×1023 atoms or molecules
When using gas laws that involve temperature, it is necessary to only use units of absolute temperature. Absolute temperature scales express absolute zero, the lowest theoretically-possible temperature, as zero on the scale. Therefore, doubling the temperature on an absolute scale represents a double in the average kinetic energy of the gas particles. The most common scale of absolute temperature is the Kelvin scale. In contrast, Fahrenheit and Celsius temperatures are not absolute and must be converted to an absolute temperature before being used for calculation.To convert C(Celsius) to K(kelvin) you need to add 273.15 to the C temperature. To convert F(Farenheit) to K you need to subtract F from 32, multiply by 5, divide by 9, then add 273.15.
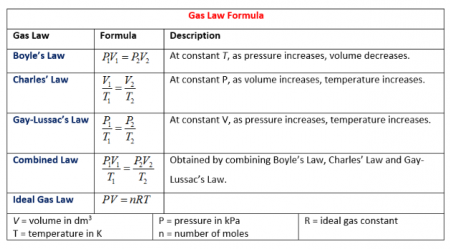
A summary of the gas laws is shown in the image to the right and the table below.
| Law | Variable quantities | Constant quantities |
|---|---|---|
| Boyle's Law | Pressure (P), Volume (V) | Temperature (T), Amount (n) |
| Charles' Law | Volume (V), Temperature (T) | Pressure (P), Amount (n) |
| Gay-Lussac's Law | Pressure (P), Temperature (T) | Volume (V), Amount (n) |
| Combined Gas Law | Pressure (P), Volume (V), Temperature (T) | Amount (n) |
| Avogadro's Law | Volume (V), Amount (n) | Pressure (P), Temperature (T) |
| Ideal Gas Law | Pressure (P), Volume (V), Temperature (T), Amount (n) | Ideal Gas Constant (R) |
Boyle's Law
Boyle's law states that for a constant temperature and amount of gas, the pressure and volume are inversely proportional. Mathematically, this can be stated as the product of the pressure and volume of the gas being a constant: [math]\displaystyle{ PV=k }[/math]
For two combinations of pressure and volume for a fixed amount and temperature of gas, the relationship may also be written [math]\displaystyle{ P_{1}V_{1}=P_{2}V_{2} }[/math]
This relationship between pressure and volume with a constant amount and temperature of gas is true because as volume decreases, gas particles are forced more closely together and the number of collisions increases, increasing pressure. The vice versa is the same, as volume increases, the gas particles are freer and there are fewer collisions, decreasing pressure.
Charles' Law
Charles' Law states that for a constant amount and pressure of gas, the volume and temperature are directly proportional. Mathematically, this can be stated as the ratio of the volume of the gas to the temperature of the gas being a constant: [math]\displaystyle{ \frac{V}{T}=k }[/math]
For two combinations of volume and temperature for a fixed amount and pressure of gas, the relationship may also be written [math]\displaystyle{ \frac{V_{1}}{T_{1}}=\frac{V_{2}}{T_{2}} }[/math]
This relationship between volume and temperature with a constant amount and pressure of a gas is true because when the temperature is increased, gas particles move faster and push on the walls of the container with more force, increasing volume as pressure has to be constant. The vice versa is the same, when the temperature is decreased, gas particles move slower and do not push on the walls of the container with as much force, making the volume decrease as well.
Gay-Lussac's Law
Gay-Lussac's Law states that for a constant volume and amount of gas, the pressure and temperature are directly proportional. Mathematically, this can be stated as the ratio of the pressure of the gas to the temperature of the gas being a constant: [math]\displaystyle{ \frac{P}{T}=k }[/math]
For two combinations of pressure and temperature for a fixed amount and volume of gas, the relationship may also be written [math]\displaystyle{ \frac{P_{1}}{T_{1}}=\frac{P_{2}}{T_{2}} }[/math]
This relationship between pressure and temperature with a constant amount and volume of gas is true because if the temperature is increased, gas particles move faster and collide with the rigid walls of the container more rapidly, increasing pressure. The vice versa is the same, when the temperature is decreased, gas particles move slower and collide with the walls of the container less rapidly, decreasing pressure.
Combined Gas Law
Any two of Boyle's, Charles', and Gay-Lussac's laws may be combined to find the combined gas law, which states that for a constant amount of gas, the ratio of the product of pressure and volume to the temperature of the gas is a constant: [math]\displaystyle{ \frac{PV}{T}=k }[/math]
For two combinations of pressure, volume, and temperature for a fixed amount of gas, the relationship may also be written [math]\displaystyle{ \frac{P_{1}V_{1}}{T_{1}}=\frac{P_{2}V_{2}}{T_{2}} }[/math]
Avogadro's Law
Avogadro's law states that for constant pressure and temperature of a gas, the amount of gas is directly proportional to the volume occupied by the gas. This can be stated as the ratio of the volume of the gas to the amount of gas being a constant: [math]\displaystyle{ \frac{V}{n}=k }[/math]
For two combinations of volume and amount of gas for fixed temperature and pressure of a gas, the relationship may also be written [math]\displaystyle{ \frac{V_{1}}{n_{1}}=\frac{V_{2}}{n_{2}} }[/math]
Many physical processes that occur at Earth's surface are assumed to occur at conditions of standard temperature and pressure (STP). These conditions are often stated to be a temperature of 0 °C and a pressure of 1 bar. Under these conditions of standard and pressure, 1 mole of gas occupies a volume of approximately 22.71 liters. However, note that these conditions apply to the present-day IUPAC definition. For a pre-IUPAC definition used before 1982, the conditions of STP were stated to be 0 °C and a pressure of 1 atm, where 1 mole of gas occupies a volume of approximately 22.4 liters under these conditions instead of 22.71. Either definition may be used on a test, but it is good to know both in case either one shows up on a test, although the pre-IUPAC definition of STP has been used on a test more frequently.
This gas law holds true for the relationship between the volume and the amount of gas with constant and fixed pressure and temperature because every gas-particle takes up space and therefore has volume, so when the amount of gas increases, the volume also increases. The same applies if the volume of a gas increases since this would mean there would have to be more gas-particles because they all take up space. If the opposite is true, the amount of gas would also decrease due to the same reason, as well as the volume decreasing as the amount of gas decreases.
Ideal Gas Law
The ideal gas law relates the pressure, volume, amount, and temperature of a gas to one another. It is given by the equation [math]\displaystyle{ PV=nRT }[/math], where [math]\displaystyle{ R }[/math] is a constant known as the ideal gas constant.
The value and units of the ideal gas constant will depend on the units used to express the variables in the ideal gas law. Some common values are given in the table below.
| Value of R | Unit of Pressure | Unit of Volume | Unit of Amount | Unit of Temperature | Unit for R |
|---|---|---|---|---|---|
| 8.314 | Pascal (Pa) | Cubic meter (m3) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{Pa*m^{3}}{mol*K} }[/math] |
| 8314 | Pascal (Pa) | Liter (L) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{Pa*L}{mol*K} }[/math] |
| 8.314×10-5 | Bar (bar) | Cubic meter (m3) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{bar*m^{3}}{mol*K} }[/math] |
| 0.08314 | Bar (bar) | Liter (L) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{bar*L}{mol*K} }[/math] |
| 0.08206 | Atmosphere (atm) | Liter (L) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{atm*L}{mol*K} }[/math] |
| 62.36 | Milimeters of mercury (mm Hg) | Liter (L) | Mole (mol) | Kelvin (K) | [math]\displaystyle{ \frac{mm Hg*L}{mol*K} }[/math] |
Combining Density and the Ideal Gas Law
The formula for density ([math]\displaystyle{ \rho=\frac{m}{V} }[/math]) and the Ideal Gas Law ([math]\displaystyle{ PV=nRT }[/math]) can be combined to solve for an unknown gas's molar mass ([math]\displaystyle{ MM }[/math]). Since molar mass is mass per moles of gas ([math]\displaystyle{ \frac{m}{n} }[/math]) and density is mass per volume of gas ([math]\displaystyle{ \frac{m}{V} }[/math]), the Ideal Gas Law can be rewritten as [math]\displaystyle{ MM=\frac{\rho RT}{P} }[/math], where [math]\displaystyle{ MM }[/math] is molar mass, [math]\displaystyle{ \rho }[/math] is density, [math]\displaystyle{ R }[/math] is the Ideal Gas Constant, [math]\displaystyle{ T }[/math] is temperature and [math]\displaystyle{ P }[/math] is pressure.
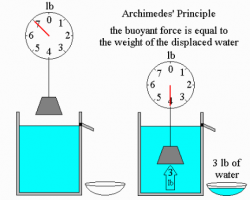
Archimedes' Principle
Archimedes' Principle states that any body completely or partially submerged in a fluid (gas or liquid) is acted upon by an upward, or buoyant, force. The magnitude of this force is equal to the weight of the fluid displaced by the body.
If the object is completely submerged, the volume of displaced fluid is equivalent to the volume of the object. If the object is floating and is only partially submerged, the volume of displaced fluid is equal to the fraction of the object's volume below the surface of the fluid. The weight of the displaced fluid is equivalent to the magnitude of the buoyant force and may be calculated using the formula [math]\displaystyle{ F_g=ma }[/math] where [math]\displaystyle{ F_g }[/math] represents the gravitational force or weight acting on the volume of the displaced fluid, [math]\displaystyle{ m }[/math] is the mass of the displaced fluid, and [math]\displaystyle{ a }[/math] is the acceleration due to gravity, which is essentially constant at 9.8 m/s2 near Earth's surface.
In the case that an object is floating and does not rise or sink, the buoyant force on the object is equivalent in magnitude to the weight of the floating object and is opposite in direction. If the object is rising, the buoyant force must be greater than the magnitude of the weight of the floating object. If the object sinks, the buoyant force has a lesser magnitude than the weight of the floating object; however, the buoyant force will reduce the sensible weight of the object, as shown in the diagram to the right.
Base and Derived Units
Some of the units used in this event are derived units, some are base units, and some units are coherently derived, but considered base SI units. Just below this is a table of the base units, including those that are coherently derived. Below that table is a table of some of the derived units.
| Name | Unit Symbol | Quantity Measured |
|---|---|---|
| cubic meter | m3 | volume |
| cubic meter per mole | m3/mol | molar volume |
| kelvin | K | temperature |
| kilogram | kg | mass |
| kilogram per cubic meter | kg/m3 | mass concentration |
| kilogram per cubic meter | kg/m3 | mass density |
| kilogram per square meter | kg/m2 | surface (area) density |
| meter | m | length |
| meter per second | m/s | velocity, speed |
| meter per square second | m/s2 | acceleration |
| mole | n | amount of gas |
| moles per cubic meter | mol/m3 | mole concentration |
| square meter | m2 | area |
| Name | Unit Symbol | Quantity Measured | Unit in base SI units | Unit in other SI units |
|---|---|---|---|---|
| degrees Celsius | °C | temperature | K - 273° | |
| joule | J | energy, work, heat | kg*m2*s-2 | N*m, Pa*m3 |
| newton | N | force, weight | kg*m*s-2 | |
| pascal | Pa | pressure, stress | kg*m-1*s-2 | N/m2 |
Reference
Glossary
| Term | Definition |
|---|---|
| Archimedes' principle | A statement that any body submerged in a fluid is subject to an upward buoyant force that has a magnitude equal to the weight of the fluid displaced by the body. |
| Area density | The amount of mass per unit surface area. It is usually represented by the Greek letter rho with a subscript A ([math]\displaystyle{ \rho_{A} }[/math]). |
| Avogadro's law | A gas law which states that for a constant temperature and pressure of a gas, the volume occupied by the gas is directly proportional to the amount of gas. |
| Boyle's law | A gas law which states that for a constant temperature and amount of gas, pressure and volume are inversely proportional to each other. |
| Charles' law | A gas law which states that for constant pressure and amount of gas, temperature and volume are directly proportional to each other. |
| Combined gas law | A gas law resulting from a combination of any two of Boyle's, Charles', or Gay-Lussac's laws. States that for a constant amount of gas the ratio of the product of the pressure and volume to the temperature is a constant. |
| Concentration | An expression of the amount of one substance that is present in a given amount of a mixture of substances. |
| Density | The amount of a substance per unit volume or area. See also mass density. |
| Gay-Lussac's law | A gas law which states that for a constant volume and amount of gas, pressure and temperature are directly proportional to each other. |
| Ideal gas law | A gas law relating the pressure, volume, amount, and temperature of a gas to one another. |
| Mass density | The mass of a substance per unit volume. It is usually represented by the Greek letter rho ([math]\displaystyle{ \rho }[/math]). May also be referred to simply as density. |
| Mixture | A collection of two or more distinct substances. |
| Mole | A unit for expressing an amount of substance, equal to 6.022×1023 atoms or molecules. |
| Number density | The number of countable objects per unit volume. It is usually represented by the Greek letter rho with a subscript N ([math]\displaystyle{ \rho_{N} }[/math]). |
| Weight | The gravitational force acting on an object. |
Metric Prefixes
| Prefix | Symbol | Multiplier |
|---|---|---|
| yotta | Z | [math]\displaystyle{ 10^{24} }[/math] |
| zetta | Z | [math]\displaystyle{ 10^{21} }[/math] |
| exa | E | [math]\displaystyle{ 10^{18} }[/math] |
| peta | P | [math]\displaystyle{ 10^{15} }[/math] |
| tera | T | [math]\displaystyle{ 10^{12} }[/math] |
| giga | G | [math]\displaystyle{ 10^9 }[/math] |
| mega | M | [math]\displaystyle{ 10^6 }[/math] |
| kilo | k | [math]\displaystyle{ 10^3 }[/math] |
| hecto | h | [math]\displaystyle{ 10^2 }[/math] |
| deca (also deka) | da | [math]\displaystyle{ 10^1 }[/math] |
| --- | --- | [math]\displaystyle{ 10^0 }[/math] |
| deci | d | [math]\displaystyle{ 10^{-1} }[/math] |
| centi | c | [math]\displaystyle{ 10^{-2} }[/math] |
| milli | m | [math]\displaystyle{ 10^{-3} }[/math] |
| micro | [math]\displaystyle{ \mu }[/math] | [math]\displaystyle{ 10^{-6} }[/math] |
| nano | n | [math]\displaystyle{ 10^{-9} }[/math] |
| pico | p | [math]\displaystyle{ 10^{-12} }[/math] |
| femto | f | [math]\displaystyle{ 10^{-15} }[/math] |
| atto | a | [math]\displaystyle{ 10^{-18} }[/math] |
| zepto | z | [math]\displaystyle{ 10^{-21} }[/math] |
| yocto | y | [math]\displaystyle{ 10^{-24} }[/math] |
Practice Tests
- azboy1910's 2020 Summer Exchange Practice Test
- azboy1910's 2020 Summer Exchange Practice Test Key
- sybisv's Density Lab Practice Test
- sybisv's Density Lab Explanation Sheet
- sybisv's Density Lab Answer Key
Additional Links
| Division B: Density Lab · Solar Power | Division C: Code Busters · WiFi Lab |