Optics
- Sections of this page may contain a large number of equations and mathematical symbols, which may take some time to properly load.
| Optics | ||||||
|---|---|---|---|---|---|---|
| Type | Physics | |||||
| Category | Lab | |||||
| Event Information | ||||||
| Latest Appearance | 2018 | |||||
| Forum Threads | ||||||
| ||||||
| Question Marathon Threads | ||||||
| ||||||
Optics is an event in Division B and Division C in the 2017 and 2018 seasons, and was previously an event during the 2011 and 2012 seasons. This event deals with geometric and physical optics, such as reflection, refraction, critical angle, electromagnetic and visible spectrum, lenses, and mirrors. Competitors for this event may bring any type of calculator and should have knowledge of SI units, as all answers will require a student to know and understand them. They must also complete the Laser Shoot, positioning mirrors around a barrier to get to a target on the wall. Some aspects of optics are similar to the event Crave the Wave, though Optics focuses on light while Crave the Wave was about waves in general.
Laser Shoot was the event's division C name from 1985 to 1989.
Introduction
Optics is a science which studies light. Optics is usually divided into two sub-fields: geometric optics and physical optics. Geometric optics studies how light travels, and includes concepts of reflection and refraction. In geometric optics, light is thought of as single rays, rather than waves since this is useful in explaining how light travels through materials such as glass or off mirrors.
Physical optics considers the wave nature of light rather than limited to single rays. This helps to explain phenomena such as interference, diffraction and refraction. Physical optics includes the visible and electromagnetic spectrum.
The Optics event for 2017 focuses mostly on geometric optics, which comprises most of the testing topics and all of the laser shoot. However, an understanding of physical optics is important for success in this event.
Geometric Optics
When waves of light (or electromagnetic waves in general) hit the boundary between two media, there are several ways that the light can react. Namely, it can reflect, refract, or be absorbed into the second medium. These will be explained below.
Reflection
The law of reflection states: The angle of incidence of a wave or stream of particles reflecting from a boundary, conventionally measured from the normal to the interface, is equal to the angle of reflection, measured from the same interface.
This may seem complex, but the scientific wording is much more complex than the idea itself. Reflection requires a "wave or stream of particles" and an interface. Light traditionally takes the former role in optics, though the idea can apply to other forms of electromagnetic radiation. For the purposes of Science Olympiad, the interface will usually be a mirror, assumed to be perfectly reflective.
Figure 1 is a convenient example of reflection. In the diagram, [math]\displaystyle{ PO }[/math] is an incoming ray of light about to reflect off a mirror. [math]\displaystyle{ OQ }[/math] is the ray after it has hit the mirror. Reflection also relies on an imaginary line, called the normal. This is an imaginary line through [math]\displaystyle{ O }[/math] and is normal or perpendicular to the mirror. The angle of incidence is the angle labeled [math]\displaystyle{ \theta_i }[/math], and the angle of reflection is the one labeled [math]\displaystyle{ \theta_r }[/math].
Based on this information, the law of reflection can be summarized by the following simple equation: [math]\displaystyle{ \theta _i = \theta _r }[/math].
Also, based on geometry, the complements to the incident and reflected angles must also be congruent.
Refraction
The speed of light is always constant when in a vacuum. However, light can change speeds while traveling through different media, such as air, water, and glass. The optical density of a medium measures how well it can transmit light. Water has a higher optical density than air, so light travels slower in water than it does in air. Note that optical density is different than the actual density of a medium.
Another way to characterize the refraction of light between media is with the index of refraction, which is useful in determining the angle at which light refracts at a boundary. The index of refraction is defined as the ratio of the speed of light in a vacuum to the speed of light in the medium. When light strikes a boundary between media at an angle, the refractive index can be calculated by using this equation: [math]\displaystyle{ n_1 sin\theta _1= n_2 sin\theta _2 }[/math] where θ(1) is the angle the light is traveling at in the first medium relative to the normal, and θ(2) is the angle the light is at after it passes through the second medium relative to the normal. The index of refraction can then be obtained by dividing the sines of the two angles.
The equation used above is known as Snell's Law. Snell's Law can be used as above, or in reverse to find the either the initial or final angle of light based on the index of refraction of both media. The refractive index of a medium is constant for every angle at which light can enter, but it is different for every wavelength of light. This is why different wavelengths of electromagnetic radiation disperse at different angles in different prisms.
In recap, Snell's Law is as follows: [math]\displaystyle{ n_1~sin~\theta _1=n_2~sin~\theta _2 }[/math]
Lenses and Mirrors
There are two main types of lenses and mirrors: convex and concave. Convex mirrors are known as diverging mirrors because the incident rays diverge upon reflection. Similarly, concave mirrors are called converging mirrors and lenses because the incident rays converge upon reflection.
In lenses, the opposite is true. Convex lenses are converging lenses, and concave lenses are known as diverging lenses. There are six types of lenses that fall under those two categories. The six lenses types are: plano-convex, plano-concave, double convex, double concave, concavo-convex, and convexo-concave.
Questions for this section will often ask students to measure the focal lengths of any of the above types of lenses and mirrors. Also, ray-tracing and properties of images are common topics on Optics exams.
An important distinction is that mirrors reflect, and lenses refract.
Vocabulary
Aperture: Describes how much light will be intercepted by the mirror.
Center of curvature: Useful when locating images, the center of the imaginary sphere upon which a curved mirror rests. The flatter the mirror, the farther away the center of curvature.
Concave mirror: Converging mirror which forms either real or virtual images which may be magnified.
Convex mirror: Diverging mirror which forms virtual images which may be magnified.
Focal length: The distance from the lens or mirror to the principal focus.
Principal axis: The main line drawn through the center of the mirror or lens upon which information such as center of curvature or principal focus is given.
Principal focus: The point where light rays parallel and close to the principal axis converge or appear to diverge.
Ray diagram: A tracing of the light rays to show where the image forms after being reflected off a mirror or refracted through a lens.
Real image: An image that can be projected onto a screen at its location, inverted relative to the object, and can be magnified.
Secondary axis: Any line drawn through the center of curvature to the mirror or lens.
Vertex: The center of a lens or mirror.
Virtual image: An image that cannot be projected onto a screen, is not inverted relative to an object, and can be magnified.
Image location in mirrors
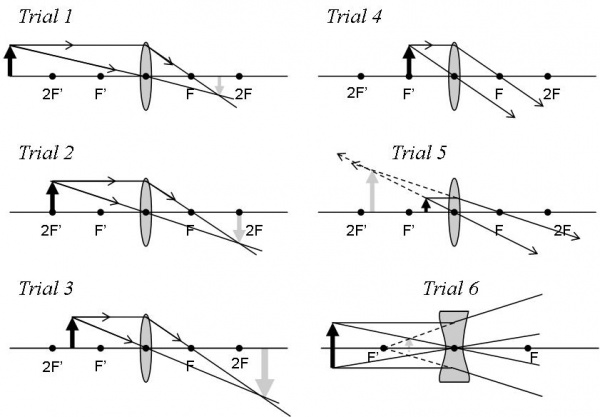
Some tests will ask for the approximate location of an image of an object reflected off a mirror. The following is a diagram of the possible locations of an image:
Trial 1: (Concave Mirror) The object is located beyond the center of curvature. The image is located between the center of curvature (C) and the principal focus (F). It is reduced in size and inverted. The image is real.
Trial 2: (Concave Mirror) The object is located at the center of curvature. The image is located at the center of curvature. It is inverted. The image is real.
Trial 3: (Concave Mirror) The object is located between the center of curvature and the principal focus. The image is located beyond the center of curvature. It is enlarged in size and inverted. The image is real.
Trial 4: (Concave Mirror) The object is located at the principal focus. No image is formed. All rays are reflected from the mirror as parallel rays.
Trial 5: (Concave Mirror) The object is located between the principal focus and the mirror. The image appears to be located behind the mirror. It is enlarged in size. The image is virtual.
Trial 6: (Convex Mirrors) All convex mirrors form a virtual image reduced in size.
NOTE: On Trial 4, the ray that does not go through the center of curvature should go through the principal focus.
Image location in lenses
Finding approximate image locations with lenses is fairly similar to finding image locations with mirrors. The only difference is that instead of having a center of curvature, the important point is listed as "2F" or twice the focal length.
Trial 1: (Convex Lens) The object is located beyond twice the focal length. The image is located between the focal length and twice the focal length on the opposite side of the lens. It is reduced in size and inverted. The image is real.
Trial 2: (Convex Lens) The object is located at twice the focal length. The image is located at twice the focal length on the opposite side of the lens. It is inverted. The image is real.
Trial 3: (Convex Lens) The object is located between twice the focal length and the focal length. The image is located beyond twice the focal length on the opposite side of the lens. It is enlarged in size and inverted. The image is real.
Trial 4: (Convex Lens) The object is located at the principal focus. Just like mirrors, no image is formed at this position. All rays are refracted from the lens as parallel rays.
Trial 5: (Convex Lens) The object is located between the principal focus and the lens. The image appears to be located behind the object on the same side of the lens. It is enlarged in size. The image is virtual.
Trial 6: (Concave Lenses) All concave lenses from a virtual image reduced in size.
Equations
Images in mirrors and lenses
[math]\displaystyle{ \frac{h_i}{h_o} = \frac{d_i}{d_o} }[/math], where [math]\displaystyle{ h_i }[/math] = image height, [math]\displaystyle{ h_o }[/math] = object height, [math]\displaystyle{ d_i }[/math] = image distance, and [math]\displaystyle{ d_o }[/math] = object distance.
Focal length of mirrors and lenses
[math]\displaystyle{ \frac{1}{f} = \frac{1}{d_o} + \frac{1}{d_i} }[/math], where [math]\displaystyle{ f }[/math] = focal Length, [math]\displaystyle{ d_o }[/math] = object distance, and [math]\displaystyle{ d_i }[/math] = image distance.
The Eye
You may be asked several questions concerning the eye. Here's a simple definition of what different parts of the eye do.
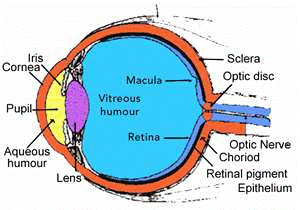
Cones: The color receptors of the eye. Cones are used more during daytime when colors are more vibrant and pronounced. There are 7 million cones in the human eye.
Cornea: The transparent part of the eye covering the iris and pupil. The cornea refracts light, providing about two-thirds of the eye's optical power.
Iris: The iris controls the size and diameter of the pupils, which serves to adjust the amount of light that enters the retina.
Lens: The lens helps to refract light and focus it onto the retina. It changes shape to adjust its focal distance so that the eye can focus on objects at different distances.
Macula The macula in the human eye is the place where light is focused by the structures in the front of the eye (cornea & lens). It takes the picture that is sent to the brain, where vision is completed. The macula provides us with the ability to read and see in great detail whereas the rest of the retina provides peripheral vision.
Optic Nerve: The optic nerve sends information from the retina to the brain so that it can be processed.
Optic Disc: The raised disk on the retina at the point of entry of the optic nerve, lacking visual receptors and so creating a blind spot.
Retina: The retina lines the inner surface of the eye and creates images based on the light passing through the eye. These images are then sent to the brain via the optic nerve.
Rods: The photoreceptors of the eye. Rods sense brightness and are favored during nighttime, when color is mostly absent and objects are viewed on a grayscale. There are 120 million rods in the human eye.
Sclera The white outer layer of the eyeball. At the front of the eye it is continuous with the cornea.
Diseases of the Eye
Myopia: nearsightedness; rays converge in front of the retina. Corrected with a diverging lens.
Hyperopia: farsightedness; rays converge behind the retina. Corrected with a converging lens
Astigmatism:irregularly shaped cornea; in some cases caused by lens (lenticular astigmatism). Corrected with eyeglasses; astigmatism causes near and farsightedness.
Cataract: Doesn’t cause pain, redness, or tearing. When the inner lens of the eye becomes darkened or opaque, the condition is called a cataract. The lens may be surgically replaced with a plastic lens. The implanted lens is of fixed focal length, so it is not capable of accommodation like the natural lens. This is usually not a major concern, because persons who develop cataracts after age 60 do not have much accommodation remaining because the inner lens has become less pliable with age.
Aphakia: Aphakia is the absence of the lens of the eye It causes a loss of accommodation, far sightedness (hyperopia), and a deep anterior chamber. Complications include detachment of the vitreous or retina, and glaucoma.
Glaucoma: Damages the optic nerve
Presbyopia: Presbyopia is a condition associated with aging of the eye that results in progressively worsening ability to focus clearly on close objects. Presbyopia is a natural part of the aging process. It is due to hardening of the lens of the eye causing the eye to focus light behind rather than on the retina when looking at close objects
Colors
Many optics tests will include sections about various forms of color mixing. It is critical to understand these concepts.
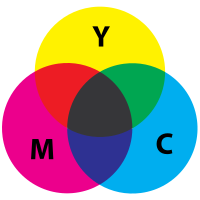
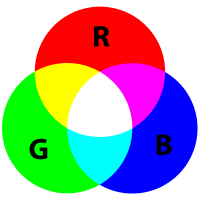
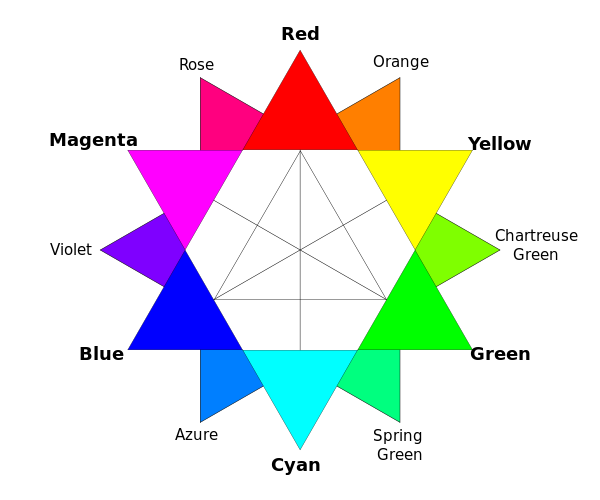
The primary colors of light are red, green, and blue. The secondary colors, meanwhile, are yellow, magenta, and cyan. Colors can be mixed in two different ways - additive and subtractive.
Additive mixing occurs in light, such as on a computer screen where red, green, and blue are mixed in different proportions to create all the other colors. Yellow is made of red and green, magenta is made of red and blue, and cyan is made of green and blue. Many optics questions will describe shining multiple lights onto a single spot, which is another form of additive color mixing.
Subtractive mixing occurs with physical dyes, like in printing or in paints. When cyan, magenta, and yellow dyes are mixed, only the primary colors in common are visible. Thus, yellow and magenta form red, cyan and yellow form green, and cyan and magenta form blue.
Another consideration is colored filters. A colored filter will transmit only the colors of light required to form that color. Therefore, a cyan filter would absorb red light, while transmitting blue and green light, and a red filter would absorb both blue and green light while transmitting red light.
Also note that this idea can be extended past primary and secondary colors:
Other Information
Energy of a Photon
[math]\displaystyle{ E=hf }[/math], where [math]\displaystyle{ E }[/math] = energy of photon, [math]\displaystyle{ h }[/math] = Planck's Constant ([math]\displaystyle{ 6.62606957\cdot 10^{−34}~~J/s }[/math]), and [math]\displaystyle{ f }[/math] = frequency.
[math]\displaystyle{ E=\frac {hc}{\lambda} }[/math], where [math]\displaystyle{ c }[/math] = the speed of light ([math]\displaystyle{ 2.998\cdot 10^{8} }[/math]) and [math]\displaystyle{ \lambda }[/math] = wavelength (in meters).
Simplified and giving the units of [math]\displaystyle{ \lambda }[/math] in microns ([math]\displaystyle{ \mu m }[/math]) and [math]\displaystyle{ E }[/math] in electron volts ([math]\displaystyle{ eV }[/math]), the above espression can be simplified and rounded to: [math]\displaystyle{ E=\frac {1.2398}{\lambda} }[/math], where 1.2398 is the rounded product of [math]\displaystyle{ h,~c,~eV (1.602\cdot 10^{-19} J), }[/math] and [math]\displaystyle{ \mu m (1\cdot 10^{-6}) }[/math]. This allows much greater convenience assuming the correct units are used.
Laser Shoot
- The information in this section is based on the 2017 rules.
Half of the score will be based on the Laser Shoot, which is the lab section of the Optics event. Points are awarded for accurately deflecting a laser beam to a target point, and for using multiple mirrors to do so.
Objective
Competitors will use up to 5 mirrors to reflect a laser around a barrier/barriers to get as close as possible to a target point. 25 points will be awarded based on the accuracy of the laser, and the other 25 points will be awarded based on the mirrors used to reflect the laser - 4 points each for the 5 movable mirrors, and 5 points for successfully using the fixed barrier mirror.
Setup
The Laser Shoot takes place in the Laser Shoot Setup, or LSS. This setup must be the same for all teams. The LSS is enclosed by a rectangular field of approximately 35 cm by 56 cm, with an open top such that students can place and adjust mirrors. A laser is placed at the center of one of the short sides, such that the laser beam is normal to the wall. On the opposite wall, a Target Point is marked at the same height as the laser, although not necessarily at the center of the wall. In both divisions, a barrier must be placed at any point along the centerline (the unrestricted path of the laser) of the LSS, such that the barrier is tall enough to block the laser and mirrors can feasibly placed to deflect the laser beam. In Division C, two additional barriers are placed at any position in the LSS. In Division B, the barrier must have a mirror attached to one side, while in Division C, any of the barriers may hold the mirror.
Procedure
- The competitors are not allowed to adjust the barrier(s) in any way. They are also not allowed to touch the laser.
- The event supervisor will provide 5 mirrors, with the front surfaces covered, which will be used to reflect the laser around the barrier(s) and towards the Target Point.
- The event supervisor must also provide a covered mirror attached to one of the barriers, which can also be used to reflect the laser for additional points.
- The competitors will be allotted 4 minutes to setup their mirrors. Once 4 minutes elapse or the competitors remove the first mirror cover, they will no longer be allowed to adjust the mirrors.
- Competitors must sign a form saying that they agree with the results.
Templates
Templates are often used in the Laser Shoot, and can range from basic graph paper to complicated printed templates. These templates must be able to accommodate the barrier mirror, whether they have a hole in the center or are split into multiple parts. Also, they must fit into the LSS box.
Templates vary in complexity, depending on how many points competitors are attempting to achieve. A simple 45 degree concept utilizing 4 mirrors can be very simple and accurate, but sacrifices 9 points of the lab score. A more complex design can be used to enable use of a fifth mirror, gaining an additional 4 points. Some templates may also be designed to use the barrier mirror, but require more fine-tuning as not all angles will be known beforehand.
Scoring
- Mirror score: (Number of mirrors hit) x 4 points, up to 20 points
- Barrier score: 5 points if the barrier mirror is also hit
- Accuracy score: 25 - (Distance from the Target point in mm/10)
The final lab score is the sum of the mirror, barrier, and accuracy score for a maximum of 50 points. The test also counts for 50 points, for a total of 100.
Resources
- Optics resources created by hftf
- HyperPhysics - Light and Vision
- Optics 2018 Sponsorship Resource Page - College of Optics and Photonics, University of Central Florida
| Division B: Helicopter · Model This · Optics | Division C: Helicopter · Protein Modeling · Sumo Bots |