Reach for the Stars
| Reach for the Stars | ||||||||
|---|---|---|---|---|---|---|---|---|
| Type | Earth Science | |||||||
| Category | Study | |||||||
| Event Information | ||||||||
| Latest Appearance | 2021 | |||||||
| Forum Threads | ||||||||
| ||||||||
| Question Marathon Threads | ||||||||
| ||||||||
| Official Resources | ||||||||
| Website | www | |||||||
| Division B Results | ||||||||
| ||||||||
Reach for the Stars is a Division B event for the 2020-2021 season. This event rotates every two years with Solar System. It was previously an event during the 2011-2012 season, 2012-2013 season, 2015-2016 season, 2016-2017 season, and 2019-2020 season.
2020-2021 Rules
Each team is allowed to bring 2 double-sided 8.5" x 11" sheets of notes and two non-programmable, non-graphing calculators. You are allowed to put anything on this paper, such as text, illustrations, tables, and pictures. Teams may also be asked to bring a clipboard and red filtered flashlight, mainly in the event that Reach for the Stars is run in a planetarium.
Part I
In Part I, students are asked to identify a specified list of stars, constellations, and deep sky objects (DSOs), which may appear on star charts, HR diagrams, planetariums, or other forms of display. Teams must also be knowledgeable about the evolutionary stages of the stars and deep sky objects on the list.
For lists of this year's and past years' stars and objects, see the Star and DSO list.
Star Charts
During competitions, the star charts given can be from any location on Earth, in any season and at any time of the night. Therefore, it is crucial to be able to recognize stars and constellations in any orientation.
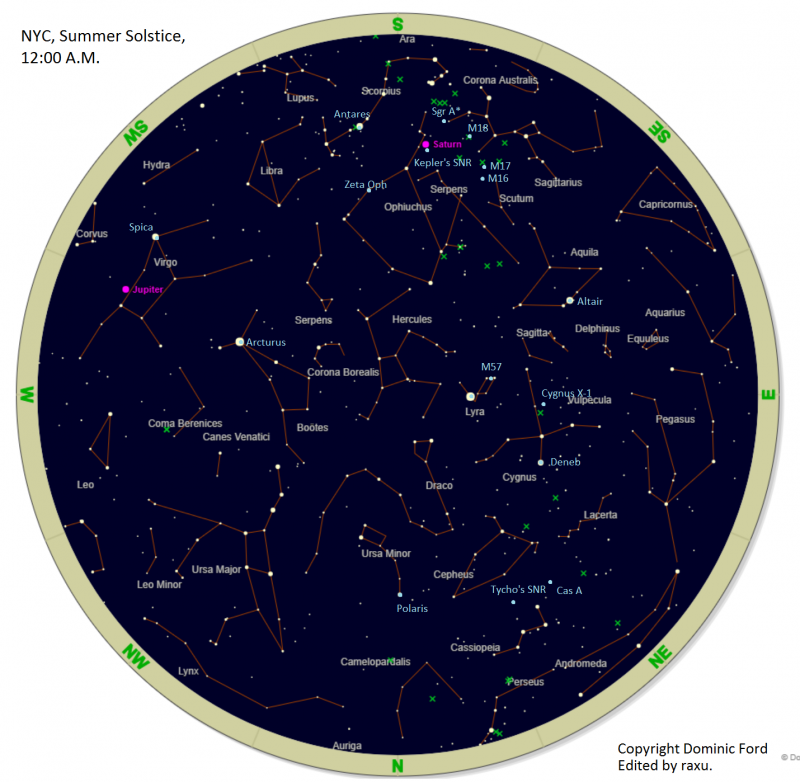
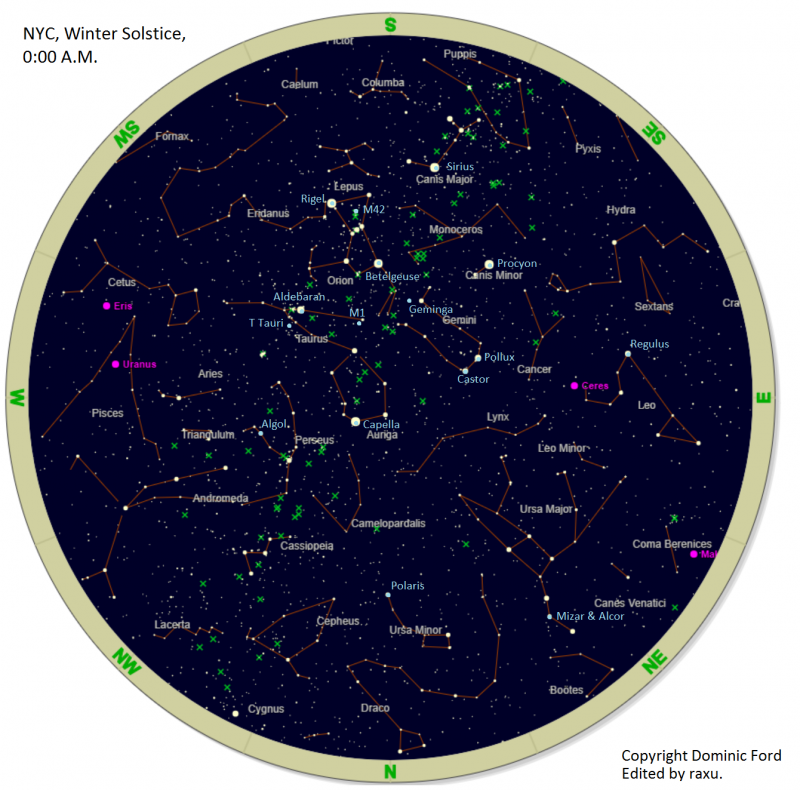
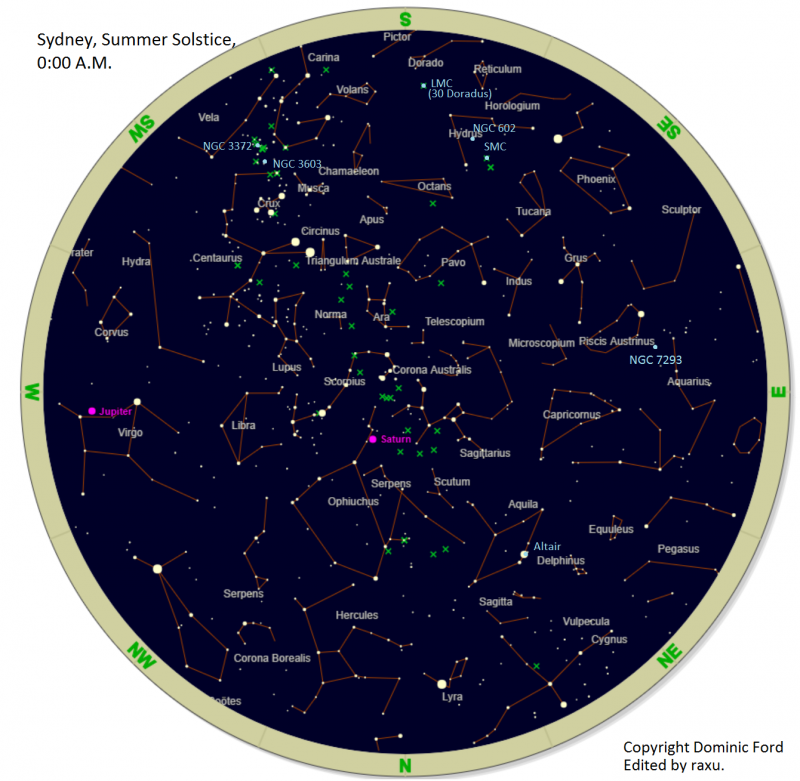
Below are three star charts that together cover all Stars and Deep Sky Objects from the 2017 Reach for the Stars list. Mizar and Alcor are binary systems, while 30 Doradus is in the Large Magellanic Cloud.
ExpandNYC, Summer Solstice
|
ExpandNYC, Winter Solstice
|
ExpandSydney, Summer Solstice
|
Identification Tips
- Go outside and look at the night sky. This is a great way to learn the constellations. Look up into the sky and use a star chart to find constellations and stars. Doing this even a few times a month really pays off.
- Flash cards with the constellation/star/DSO on the front and its name on the back can really help. Make different flash cards with different information on the front page, such as constellation shape, location on a star chart, or pictures.
- Another tip is to use Quizlet, which is great for studying constellations, stars, and DSO's, and may show images that could be seen on a test.
- When working with star charts or looking at the night sky, many have found it very helpful to relate easy-to-find constellations such as Orion or Ursa Major (Big Dipper) to the constellations around them. This guides you to the constellation via others, rather than having to rely only on the shape. It may be helpful to include a section on the reference sheet about finding constellation you have trouble with on a sky chart. Common "pathways" include:
- Using the handle of the Big Dipper asterism to find Bootes and Arcturus, and Virgo and Spica, and using the cup to find Ursa Minor and Polaris.
- Using Orion to find Taurus, then Auriga, and then Gemini, Perseus, and Andromeda. Cassiopeia is also good for finding Perseus and Andromeda.
- Looking for the Winter Triangle (Betelgeuse, Sirius, Procyon) and Summer Triangle (Vega, Altair, Deneb) to find those stars and constellations.
- Using the Zodiac constellations, which are close to each other around the Ecliptic. This helps with Leo, Virgo, Scorpius, Sagittarius, and Ophiuchus (along with Serpens) especially, but also Gemini and Taurus.
- Sometimes, the test will use a StarLab or planetarium for the identification portion. Put some time in to familiarize yourself with how the skies look on it. This will help reduce confusion on the identifications and reduce the amount of time spent on those questions.
- There is always a chance that a bad star map may be used, so make sure to get accustomed to using less-than-clear maps and images.
- In general, the brightest star (lowest apparent magnitude) in a constellation is denoted Alpha, and second brightest Beta, and so on. After the Greek alphabet has been used up, numbers are used - 1 is dimmer than Omega but brighter than 2. However, there are exceptions: Betelgeuse (Alpha Orionis) usually appears dimmer than Rigel (Beta Orionis), and Castor (Alpha Geminorum) appears to be dimmer than Pollux (Beta Geminorum).
- While identification is not the only part of this event, it is a good way to begin preparing. For the rest of the event, see Part II. A good resource is Astronomy Today.
Part II
In Part II, students are asked to complete tasks relating to a topics in Astronomy. Students should know about the general characteristics of stars, galaxies, star clusters, etc. Furthermore, students should be able to find a star's spectral class, surface temperature, and evolutionary stage (protostar, main sequence, giant, supergiant, white dwarf) given its position on the Hertzsprung-Russell diagram, or place the star on the diagram given these information. Finally, students should be familiar with basic Astrophysics knowledge such as Luminosity scales and relationships, temperature relationships, flux, and distance measures. For a more in-depth study of astrophysics, please see the Astronomy page, but Reach for the Stars is unlikely to reach the level of complexity of the C Division event.
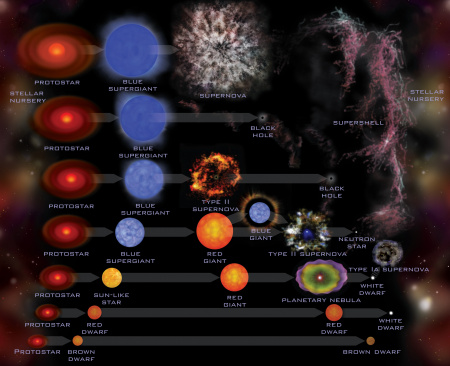
Stellar Evolution
This section gives a brief overview of Stellar Evolution. A more detailed discussion of stellar evolution may be found on the Astronomy/Stellar Evolution page, and other associated pages.
Formation
Star formation starts in a dense interstellar cloud - a dark dust cloud or a molecular cloud. After some instability or disturbance, the cloud collapses under its own gravity, and form clumps of matter. As the clump contracts further, its density grows, its temperature rises, and the clump becomes a protostar.
The protostar contracts further and evolves onto the main sequence following the Hayashi track. It enters main sequence when nuclear fusion begins.
Main Sequence
Stars spend the majority of their lives on the main sequence. Heavier stars spend significantly less time on the main sequence because their rate of fusion is much higher. The radiation pressure from fusion balances with gravity, and the star is in hydrostatic equilibrium.
Lower-mass stars fuse Hydrogen into Helium mostly using the proton-proton (p-p) chain. This process starts at around [math]\displaystyle{ 4\cdot10^6 }[/math] Kelvins.
Higher-mass stars fuse Hydrogen into Helium mostly using the CNO Cycle. The CNO cycle uses Carbon, Nitrogen and Oxygen as catalysts, and is dominant in temperatures higher than [math]\displaystyle{ 17\cdot10^6 }[/math] Kelvins.
Post-Main Sequence, Low Mass
When the core of a low-mass star is depleted of Hydrogen, nuclear fusion subsides because Helium fusion occurs at much higher temperature. The core contracts, and the heat generated by the contraction heats up the outer layers, creating a Hydrogen-burning shell. The star also expands and becomes a red giant.
The core then reaches high enough temperature for helium fusion. For a moment, helium is fused rapidly in a runaway condition known as the helium flash, but then subsides as the star enters the horizontal branch.
When a low-mass star runs out of helium, it does not heat up high enough to fuse Carbon into heavier elements, and fusion stops. The core compresses further into a white dwarf, when electron degeneracy pressure prevents it from compressing further, and the outer shell expands into a planetary nebula, heated up by the white dwarf core. The white dwarf slowly cools and becomes a black dwarf.
Post-Main Sequence, High Mass
When the core of a high-mass star is depleted of Hydrogen, the star expands into a red supergiant. Its luminosity stays roughly the same because the temperature of the outer shell decreases. Helium starts fusing into carbon without a runaway process, so there is no helium flash.
A high-mass star is able to fuse elements up to iron, at which point further fusion consumes energy instead. The iron core contracts further. The star explodes in a bright core-collapse supernova. Then, either electrons combine with protons and the core is made of neutron degenerate matter - a neutron star - or the core contracts further into a black hole. Neutron stars rotate very rapidly and have very strong magnetic fields. Some neutron stars are called pulsars and magnetars for their additional features.
Spectral Classification
Harvard Spectral Classification
There are 7 spectral Classes (O,B,A,F,G,K,M). This order is based on decreasing surface temperature. A Class stars have the strongest Hydrogen lines, while M-Class stars have the weakest hydrogen lines. Each class is then subdivided into 10 subdivisions (0-9).
The following is a table with properties of each of the spectral classes.
| Type | Temperature (Kelvin) | Color | Hydrogen |
|---|---|---|---|
| O | 30,000-60,000 | Blue | Weak |
| B | 10,000-30,000 | Blue-White | Medium |
| A | 7,500-10,000 | White | Strong |
| F | 6,000-7,500 | White | Medium |
| G | 5,000-6,000 | Yellow | Weak |
| K | 3,500-5,000 | Yellow-Orange | Very Weak |
| M | 2,000-3,500 | Red | Very Weak |
The following is the class of each of the stars on the list:
- Class O: Zeta Ophiuchi
- Class B: Rigel, Spica, Regulus, and Algol
- Class A: Vega, Sirius A, Deneb, Altair, Castor, Mizar, and, Alcor
- Class F: Procyon, and Polaris
- Class G: Capella
- Class K: Arcturus, Aldebaran, and Pollux,
- Class M: Betelgeuse, and Antares
There are also L, T, and Y for brown dwarfs, which are generally not considered stars. They can fuse Deuterium and Lithium, but are not hot enough to fuse Hydrogen into Helium via the Proton Proton Chain.
Yerkes Spectral Classification
The Yerkes Spectral Classification is based on luminosity and temperature. It is also known as luminosity classes. There are seven main luminosity classes:
- Type Ia: Bright Supergiants
- Type Ib: Normal Supergiants
- Type II: Bright Giant
- Type III: Normal Giant
- Type IV: Sub-Giants
- Type V: Main Sequence
- Type VI: Sub-Dwarf
- Type VII: White Dwarf
There is also Type 0, for hypergiants. However, these are exceedingly rare; examples include VY Canis Majoris, the Pistol Star, and R136a1.
Radiation Laws
NOTE: This and the following section contain some algebra. If you are not yet comfortable with algebra, you can still read these sections for the theoretical concepts.
The radiation laws show relationships between stellar temperature, radius, and luminosity. All three laws are regarding black bodies, ideal objects that absorbs all incoming radiation. Stars, with little incoming radiation, are often approximated as black bodies to simplify calculations.
Both Wien's Law and Stefan's Law are proportionality statements, that a change in one quantity is always accompanied by change in other. These can be turned into equations by introducing a constant known as a proportionality constant. The proportionality statement [math]\displaystyle{ y\propto x }[/math] denotes that if [math]\displaystyle{ x }[/math] changes by a factor [math]\displaystyle{ k }[/math] (here, "k" is just an arbitrary variable), [math]\displaystyle{ y }[/math] also changes by [math]\displaystyle{ k }[/math]. However, it does not mean that the values are equal to each other: a proportionality constant needs to be added.
At Division B it is unlikely that one will perform calculations with these laws, but general questions regarding these laws, such as the proportionality, may be asked. For details about calculations with these laws, visit the Astronomy page.
Wien's Law
Wien's displacement law states that the wavelength where a black-body emits most of its radiation is inversely proportional to the temperature. In other words, as the temperature of a star increases, the wavelength at which the star emits most of its radiation decreases. As a proportionality statement,
where [math]\displaystyle{ {\lambda}_{max} }[/math] is the wavelength of maximum output of radiation from an object and [math]\displaystyle{ T }[/math] is Temperature. For example, if the temperature of a star is multiplied by 2, the wavelength of maximal radiation would be divided by 2.
In order to use this as a normal equation, a proportional constant needs to be added. In this case, [math]\displaystyle{ b=2900\mu m\cdot K }[/math] known as the Wien's displacement constant, is used. Then,
where [math]\displaystyle{ \lambda_{max} }[/math] is in micrometers and [math]\displaystyle{ T }[/math] is in Kelvin. The equation form of the law is more common in Division C, and is unlikely to appear in this event.
Stefan-Boltzmann's Law
The Stefan-Boltzmann Law states that the total energy emitted from a black-body per unit surface area is proportional to the fourth power of its temperature. In equations,
where [math]\displaystyle{ j^* }[/math] is the total energy emitted per unit area and [math]\displaystyle{ T }[/math] is Temperature. For example, if the temperature of a star is multiplied by 2, the total energy emitted per unit surface area would be multiplied by [math]\displaystyle{ 2^4=16 }[/math].
The proportional constant for this equation is the Stefan–Boltzmann constant [math]\displaystyle{ \sigma=5.67\cdot 10^{-8}\mathrm{W/m}^2\mathrm{K}^4 }[/math]. Therefore, [math]\displaystyle{ j^*=\sigma T^4 }[/math], where [math]\displaystyle{ j^* }[/math] is in Watts per square meter, and [math]\displaystyle{ T }[/math] is in Kelvin. Again, the equation form is unlikely to appear.
Since all black-bodies we encounter are considered to be spheres, they have surface area [math]\displaystyle{ A=4\pi R^2 }[/math], where [math]\displaystyle{ R }[/math] is the radius of the object. The luminosity ([math]\displaystyle{ L }[/math]) of a star, which is the total energy emitted from the star, is the product of [math]\displaystyle{ j^* }[/math] and [math]\displaystyle{ A }[/math]. Therefore, since [math]\displaystyle{ A\propto R^2 }[/math] and [math]\displaystyle{ j^*\propto T^4 }[/math], we end up with the following relationship:
Stefan's Law relates three different quantities of a star: its luminosity, temperature and radius. Notice that an increase in temperature will have much more of an effect on luminosity than an increase of the same factor in radius, since temperature is raised to the fourth power.
Putting all of the constants back into the relationship to make it an equation again, [math]\displaystyle{ L=4\pi {R}^{2}\sigma {T}^{4} }[/math], with [math]\displaystyle{ L }[/math] in Watts, [math]\displaystyle{ R }[/math] in meters, and [math]\displaystyle{ T }[/math] in Kelvin. Again, questions requiring the use of this specific equation are beyond the scope of this event.
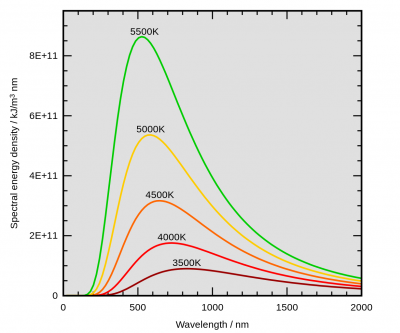
Planck's Law
Planck's Law states that a hotter blackbody emits more energy at every frequency than a cooler blackbody. The equation form of the law is complicated, while on a radiance vs. temperature graph the law states that the curve for a hotter blackbody never dips below that of a cooler one.
Magnitude and Luminosity Scales
The luminosity of a celestial object refers to how much radiation (visible light, infrared, x-ray, etc.) it emits per unit time. Luminosity is measured in Joules per second or Watts. The luminosity, of the sun, for example, is [math]\displaystyle{ L_\odot=3.846\cdot 10^{26} }[/math] watts. Magnitude scales are different methods to express luminosity.
Apparent Magnitude
The apparent magnitude, denoted by [math]\displaystyle{ m }[/math], denotes the brightness of a celestial object as seen by an observer on Earth. The brighter an object appears, the lower its apparent magnitude. It is a logarithmic scale, not a linear scale, which means that a small decrease in magnitude results in a much greater increase in luminosity. For example, an object with apparent magnitude 5 less than that another would seems 100 times more luminous. Logarithms are very advanced for Division B, but at the very least, it is important to know that a small change in magnitude represents a much larger change in luminosity.
For example, the sun has apparent magnitude of -26.74, while Deneb has apparent magnitude of 1.2. Because of this, the sun seems [math]\displaystyle{ 100^{(1.2+26.74)/5}\approx 150 \text{ billion} }[/math] times brighter than Deneb! Apparent magnitude depends on both the luminosity of the object and its distance from Earth: while Deneb is more luminous than the sun, to an observer on Earth it is dimmer because it is farther away.
The system of apparent magnitude originated from Greece, where the brightest stars in the night sky were of first magnitude ([math]\displaystyle{ m=1 }[/math]), while the faintest to the naked eye were of sixth magnitude ([math]\displaystyle{ m=6 }[/math]). The system was formalized and extended beyond 1 to 6 in 1856 by N. R. Pogson.
Absolute Magnitude
The absolute magnitude, denoted by [math]\displaystyle{ M }[/math], denotes the brightness of a celestial object as seen by an observer 10 parsecs (about 32.6 light years) away from the object. Similarly, an object with absolute magnitude 5 less that of another would be 100 times more luminous. The absolute magnitude is basically another way of expressing the luminosity of the object. Scientists often consider the absolute bolometric magnitude [math]\displaystyle{ M_b }[/math] of an object, meaning that its radiation is being measured across all wavelengths.
For example, the sun has absolute magnitude 4.83, while Deneb has absolute magnitude -8.38. This means Deneb is [math]\displaystyle{ 100^{(4.83+8.38)/5}\approx 192\text{ thousand} }[/math] times more luminous than the sun. A typical Type Ia supernova has an absolute magnitude of about -19.3.
Inverse Square Law
The inverse square law says that a certain quantity is inversely proportional to the square of the distance relating to that quantity. In this case, "inversely proportional" means that an increase of one number causes a decrease in the other number. For example, suppose an astronomer measures a star of some intensity ([math]\displaystyle{ I_1 }[/math]) at a certain distance ([math]\displaystyle{ D }[/math]) from the source. By the inverse square law, we have the following proportion:
This law also applies to Newton's Law of Gravitation. The law states that:
where [math]\displaystyle{ m_1 }[/math] and [math]\displaystyle{ m_2 }[/math] are the masses of two objects, [math]\displaystyle{ r }[/math] is the distance between the two objects, and [math]\displaystyle{ G }[/math] is a special constant called Newton's gravitational constant. Since most objects in space are very far away from each other, the bottom part of the fraction is much larger than the top part of the fraction, so the law can be approximated for most far-apart objects by [math]\displaystyle{ F\approx\frac{1}{r^2} }[/math].
The law also applies to the electrostatic force and the intensity of sound wave in a gas.
Distance Modulus
Distance modulus is a way to relate the absolute and apparent magnitudes of objects with the distance between them. The distance modulus equation is as follows:
where [math]\displaystyle{ m }[/math] is apparent magnitude, [math]\displaystyle{ M }[/math] is the absolute magnitude, and [math]\displaystyle{ d }[/math] being the distance to the object in parsecs. This equation uses a logarithm, which will more often than not be outside the scope of the Division B event, but for a brief tutorial on logarithms, please see this link. A different way of expressing this equation is:
For example, the Supernova SN 2011fe had peak apparent magnitude of [math]\displaystyle{ m=+9.9 }[/math], while its absolute magnitude is about [math]\displaystyle{ M=-19.3 }[/math]. Therefore, the supernova is
away from Earth, close to the experimental value of [math]\displaystyle{ 6.4\pm0.5\text{ Mpc} }[/math].
This relationship can be found by using the laws that were discussed earlier. By the inverse square law, an observer 10 times as far as another from the same object would see the object as being 100 times less bright, and so they would mark it as having an apparent magnitude of 5 more than the other observer. Since absolute magnitude is the "apparent magnitude" of an observer 10 parsecs away, the first distance equation can be found by relating all of these factors. This is helpful to know in case theoretical questions are asked about the distance modulus relationship, but tests are unlikely to ask about the fine details for Reach for the Stars.
Galaxies
There are three main types of galaxies: Spiral, Elliptical, and Irregular. However, in the 2013 rules, there are no galaxies on the list. Nevertheless, galaxies are an important part of astronomy, so here is a brief background on the types of galaxies.
Spiral Galaxies
Spiral Galaxies are named so because they have prominent spiral arms and a central "galactic nucleus" or central bulge.
Spiral Galaxies also have a very large rate of star formation in the spiral arms of the galaxy. Also, almost all spiral galaxies have a galactic halo that surrounds the galaxy. These halos contain stray stars and globular clusters. It is also theorized that many spiral galaxies have supermassive black holes at the center of the galaxy. Our own galaxy, The Milky Way, is a spiral galaxy, and is also theorized to have a supermassive black hole at its center, called Sgr A*. There is also a sub-division of spiral galaxies, known as barred-spiral galaxies. Barred-spirals have a central bar, and then have spiral arms shooting off at each end of the bar.
Spirals are classified by presence of a central bar and how tightly the rings are wound.
| Sa | tightly wound, no central bar |
|---|---|
| Sb | moderately tightly wound, no central bar |
| Sc | loosely wound, no central bar |
| Sd | very loosely wound, no central bar |
| SBa | tightly wound, central bar |
| SBb | moderately tightly wound, central bar |
| SBc | loosely wound, central bar |
| SBd | very loosely wound, central bar |
The spiral galaxies on the list for 2020 are:
- M31 Andromeda Galaxy (in Andromeda)
- M101 Pinwheel Galaxy (in Ursa Major)
- NGC 4676 Mice Galaxies (in Coma Berenices)
- NGC 4038/4039 Antennae Galaxies (in Corvus)
Lenticular Galaxy
Lenticular Galaxies are intermediate between spiral and elliptical Galaxies, they contain a large scale disk, but do not have spiral arms. Like Elliptical Galaxies, they contain older Stars and have a low rate of star formation.
The Lenticular Galaxies on the list for 2020 are:
- M104 Sombrero Galaxy (in Virgo)
- NGC5128 Centaurus A (in Centaurus)
Elliptical Galaxy
Elliptical Galaxies appear just like they sound- they are elliptical/ spherical. Elliptical Galaxies contain mostly old Population II stars, and also, they have a very low rate of star formation because there is barely any interstellar matter in elliptical galaxies. There is the least amount of Elliptical Galaxies in the known Universe. Also, they are classified by how spherical they are, with E followed by a number from zero to seven. Zero indicates perfectly spherical; seven indicates the extremely elongated and cigar-shaped.
The Elliptical Galaxies on the list for 2020 are:
- M60 (in Virgo)
- NGC 4555 (in Coma Berenices)
Irregular Galaxies
Irregular also appear just how they sound- they are without a definite shape. They are normally formed by Spiral or Elliptical Galaxies that have been deformed by different forces- such as gravity. They contain a lot of interstellar matter. There are distinctions between "normal" irregular galaxies - with no hint of shape - and peculiar galaxies, that have some hint of form - usually, they were bent out of shape by outside forces or became violently active.
The Irregular Galaxies on the list for 2020 are:
- Large Magellanic Cloud (in Dorado and Mensa)
- Small Magellanic Cloud (in Tucana)
Photo Gallery
Sample Tests
- Identification practice: Reach for the Stars Test (2009)
- RFTS Test and Pic Sheet for the test
- Also be sure to check out the Reach for the Stars Test Exchange.
Useful Resources and Links
- An Example of a Reach For The Stars Study Sheet
- Another Example of a Reach For the Stars Guide Sheet (2007)
- Astronomy Today by Eric J. Chaisson
- Foundations of Astronomy by Michael A. Seeds
- Photo index of the Chandra Observatory (Good for DSOs)
- STSCI Office of Public Outreach
- New York Coaches Conference
- Astronomy Picture of the Day
- Hertzsprung-russell diagram study
- Astronomy blog, by scioly.org's own AlphaTauri, syo_astro, and foreverphysics