Quantum Quandaries
This page is incomplete. |
| Quantum Quandaries | |
|---|---|
| Type | Physics |
| Category | Study |
Quantum Quandaries is a Division C event last run as a trial event at the 2006 National Tournament, 2016 MIT Invitational, and 2022 Yale Invitational. It relates to quantum mechanics and other areas in modern physics, such as nuclear and particle physics.
Competition
The event is run as multiple stations, with a portion of those stations involving laboratory tasks (at the state and national level). Students are allowed to bring any type of calculator, along with two 8.5"x11" sheets of notes (front and back). Other notes may be provided at the event coordinator's discretion.
Quantum Mechanics and Technology
Principles
Observables and Measurement
Quantities or properties of systems that we can measure, such as charge and momentum, are referred to as observables or operators. Measurement, in quantum mechanics, refers to any interaction that reveals information about a particle.
Note: This definition of measurement comes from what is known as the Copenhagen interpretation, which is a viewpoint on the unknown mysteries of quantum mechanics (including what measurement means physically, not just mathematically). Therefore, the interpretation is up for debate, but it remains one of the most popular and most widely taught interpretations since its development in the 1920s.
Uncertainty Principle
One of the main principles of quantum mechanics is the idea that nothing is certain. This is reflected in Heisenberg's uncertainty principle, which states that you can only measure up to a certain point position and energy. If you measure with an arbitrary amount of accuracy position, you are unable to determine arbitrarily energy, and vice versa. Thus, position and momentum are referred to as noncommutative observables . However, the uncertainty principle can refer to any relationships between noncommutative operators. Mathematically, this occurs because the standard deviations of the two measured properties are inversely related, meaning that decreasing the standard deviation of one increases the standard deviation of the other.
It is very important that one does not confuse the uncertainty principle with the Observer effect, which states that attempting to measure a system will inevitably affect some of its properties, essentially referring to experimental error. Although such error may be present in experiments, measurements under the uncertainty principle can include any interaction that reveals information about a particle, even just involving other particles.
Wave-Particle Duality
One other principle is wave-particle duality. This was discovered in part by Young's double-slit experiment and states that photons can be both a wave and a particle, depending on what state it has been detected at.
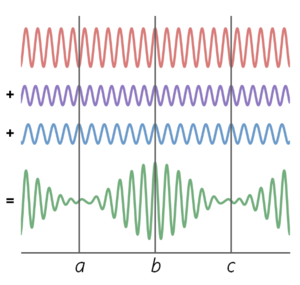
According to the Copenhagen Interpretation of quantum mechanics, the wave-particle duality does not mean that particles are also waves like ripples on water, spreading in every direction. Instead, these waves are actually wave packets of probability. To dissect this statement, we first look at the term "wave packet." If you were to think of a sinusoidal wave, you would imagine one with constant amplitude and frequency, traveling endlessly in either direction. A wave packet is instead a small portion of a sinusoidal wave that may have varying amplitude and frequency.
As shown in the image above, the wave packet is mostly localized (that is, most of the amplitude is distributed closely together with marginal amounts on the edges). In addition, the amplitude and frequency vary. This wave packet is created by adding the amplitudes of multiple sinusoidals. When the sum of the amplitudes is equal to (or relatively close to) 0, it is referred to as destructive interference. When the amplitudes add up, resulting in a greater amplitude in the wave packet, it is referred to as constructive interference. This combination of waves is actually an example of superposition, where a single wave is formed of multiple waves.
It is very important to note that, around the edges of this image, the probability amplitudes begin to increase again. This is a result of the fact that the superimposed waves are periodic and infinite, so any interference patterns are bound to be periodic as well. However, these edge values can be largely ignored as, realistically, the wave function is much more complex than depicted (to be precise, the superposition shown above is considered discrete, whereas accurate models use continuous superposition). This representation of a wave packet is only useful for showing interference patterns using simple waves. With an accurate model, the probabilities outside of the central region are very close to 0 and usually not depicted.
Returning to the original statement (that waves are wave packets of probability), we can now look at the probability portion. This actually says that, unlike physical waves where molecules of water or another liquid move to create a wave-like shape, the amplitudes of wave packets are actually probability amplitudes spread throughout space. At each point in space (a position in the horizontal x-axis), there is an associated probability value (the y-value of the graph at that x-value) that represents the probability of the "particle" existing at that position in space. As a result, clusters such as those in the image above are strong candidates for the position of the particle, but because of uncertainty, we cannot be sure as to its exact position. This is marked by the space between points a and c, showing that there is a region of space where the particle is most likely to appear.
Photoelectric Effect
When a beam of light hits a piece of metal, photons may be ejected from its path. This is a sign of the photoelectric effect. This occurs because photons free electrons that are in the orbitals of the metal, thus allowing the photon to escape with a certain energy that correlates to the energy difference needed to free the electron. Albert Einstein was awarded the 1921 Nobel Prize in Physics for his discovery of the photoelectric effect.
Spectroscopy
Spectroscopy is the study of a system or object using its energy, wavelength, or other property related to its energy. These properties provide insight into the interactions and behavior of the system or object. In addition, objects can be uniquely identified using their spectra, such as in astronomy, where stars and other objects that emit radiation can be classified using their emission or absorption spectra.
Relativity and Cosmology
Black Holes
One special property of stars is that their entire lives and evolution can mostly be predicted solely from the mass of whatever formed them. Stars usually form from large clouds of gas called protostars which collapse from gravitational pressure to form what are known as pre-main-sequence stars. As these stars age, their initial mass determines the physics of how the evolve, including how they die. If a star has a core that has a mass above the Tolman–Oppenheimer–Volkoff limit, which is debated to be around 2-3 solar masses (i.e. 2-3 times the mass of the sun), it will collapse to form a black hole.
The Big Bang
Around 13 billion years ago, the universe began in a blast of energy called the Big Bang. The Big Bang started from an infinitesimal point of space and began to expand over time. As it cooled, particles began to form and the structure of the Universe began to become more apparent. The Big Bang can be divided into 3 main time periods.
Lorentz Transformations
According to Einstein's Special Theory of Relativity, an observer moving at a constant velocity will experience events differently than an observer at rest. To describe the differences in these observations, the Lorentz transformations can be used to transform values in one reference frame to another. The transformations only apply in inertial reference frames, i.e. ones that do not accelerate relative to another inertial reference frame. By extension, since rotation requires a change in direction of velocity, which is represented by acceleration, reference frames that are rotating relative to another reference frame are not inertial.
Twin Paradox
One paradox involved with time travel is the twin paradox. The twin paradox involves two identical twins, one that stays on Earth and one that goes on a rocket that goes at 99% the speed of light. When the astronaut goes to a certain distance and comes back, the astronaut finds that the twin on Earth has aged more. This is because in the astronaut's frame of reference, time has slowed down, while according to the twin, time has not slowed down.
Gravitational Lensing
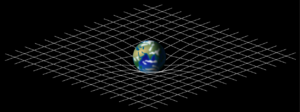
Einstein's general theory of relativity states that objects with mass cause curvature in spacetime around them, and this effect is the explanation for gravity. This curvature of spacetime is often thought of using the analogy of a bowling ball on a trampoline pulling down on the fabric, as shown in the figure below. This analogy does have downfalls, such as not explaining how the bowling ball weighs down on the trampoline without circling back to gravity, but the idea of curvature is the point here. For those who are curious, the curvature is actually caused by complex properties of the energy and momentum of the body of mass, but that is very high-level and not required knowledge for this event.
The existence of matter bends spacetime around the matter, and this spacetime gives a description of how objects move through spacetime. All objects in spacetime must follow the curvature of spacetime, and light is no exception. Thus, when light approaches near a massive object, it moves along the curvature, causing it to bend at a slight angle known as the angle of deflection. This is what makes the light look like it's flowing around the object, creating shapes like an Einstein Ring or an Einstein Cross.
Since the path of light is altered, near a massive object, the distance the light has to travel to reach the observer also changes. However, Einstein showed in his special theory of relativity that the speed of light is constant. Since the time it takes an object to travel a distance is dependent on the distance and speed, increasing distance increases the amount of time it takes for the object to travel that distance. As a result, gravitational lensing can delay some of the light traveling from a single source, making it appear multiple times in the sky. This has been observed many times in the past, proving the existence of gravitational lensing.
Nuclear and Particle Physics
Standard Model
The Standard Model allows one to correlate the forces and the particles that govern the forces. There are two main types of particles in the Standard Model: fermions and bosons.
Fermions
The fermions can be further subdivided into the quarks and leptons. Fermions are the elementary particles that are characterized by having half-integral spin i.e. spin [math]\displaystyle{ \frac{n}{2} }[/math], where [math]\displaystyle{ n }[/math] is an integer. Quarks, leptons, and all particles made out of an odd number of these are counted fermions.
Quarks
There are six types of quarks: up, charm, top, down, strange, and bottom. It is important to note that these names have no relationship with any physical properties of the particles themselves.
Leptons
The six leptons can be classified into two groups. The first group consists of the electron, muon, and tau lepton (sometimes called the "tauon" or "tau particle"). Each of these particles forms a family with a corresponding neutrino. These are the electron neutrino, muon neutrino, and tau neutrino, respectively.
Bosons
Bosons are force-carrying particles that are characterized by having integer spin i.e. spin [math]\displaystyle{ n }[/math]. They are classified into the gauge bosons, which are sometimes also called vector bosons, and the scalar bosons. The name is slightly misleading because vectors bosons are simply bosons with spin 1, and scalar bosons are bosons with spin 0. These names come from the mathematical representation of the bosons as tensors, but that is out of the scope of this event.
The graviton, a theoretical particle that is responsible for gravity, would be the first tensor boson (again, the name can be misleading). The discovery of a graviton would allow physicists to develop a theory of quantum gravity, uniting general relativity (which describes gravity) and quantum mechanics. The graviton is uniquely a boson with spin 2, so the discovery of any particle with spin 2 would also be the discovery of the graviton.
Scalar Bosons
The scalar bosons are the simplest group, as the only known scalar boson is the Higgs boson, theorized in 1964 and discovered in 2012 at the Large Hadron Collider (LHC) in Geneva, Switzerland. In fact, the LHC was primarily built to discover particles like the Higgs boson! The Higgs boson is very important because it explains why the W boson and Z boson, which should be massless, actually have a relatively large amount of mass compared to the other fundamental particles. It provides a mechanism, appropriately called the Higgs mechanism, that explains how these particles gain a mass.
To discover the Higgs boson, particle physicists at the LHC first defined certain characteristics of the particle, including its energy range, interactions, and decay products. By performing high-energy collisions, they attempted to produce excitations in the Higgs field, where an excitation represents the possible existence of a particle (read Unified Field Theory for an overview of fields). By producing an energy spike which deviated from the rest energy, they were able to detect the particle. After that, they used other observations to confirm that the particle was indeed the Higgs boson and not another particle.
Vector Bosons
The group of vector bosons/gauge bosons includes the photon, gluon, Z boson, and W boson. The photon is simply the basic unit (sometimes called the quantum, plural quanta) and force carrier of the electromagnetic field. The study of these interactions is called Quantum Electrodynamics (QED). The gluon is the mediator for the strong force between quarks, which is the force responsible for creating hadrons, e.g. protons and neutrons. The gluon also is responsible for color change, which is completely unrelated to physical color, despite its name, since light does not interact at the scale of quarks. Color change simply refers to the "color" that a quark has; gluons carry both color and anticolor, and these are exchanged between quarks. The study of the strong force and these color changes is called Quantum Chromodynamics (QCD). Lastly, the Z boson and W boson are together referred to as the "weak bosons" as they mediate the weak interaction, which is most notable for its role in beta decay of neutrons into a proton, electron, and electron antineutrino. The weak interaction is studied in the electroweak theory (EWT).
Composite Particles
Composite particles, i.e. particles that are composed of multiple particles in the standard model, can be classified as either fermions or bosons. In particular, a particle made up of an odd number of fermions would also be a fermion (since a half-integer spin times an odd number is also a half-integer spin). For example, baryons, which contain an odd number of quarks, are classified as fermions.
In addition to this, some composite particles can be classified as hadrons, meaning they are solely made up of quarks. Fermion hadrons are referred to as baryons and boson hadrons are referred to as mesons.
Baryons
A baryon is any particle that has an odd number of quarks. Protons and neutrons are examples of baryons. Protons contain two up quarks and one down quark. Up quarks have a charge of [math]\displaystyle{ +\frac{2}{3} }[/math] and down quarks have a charge of [math]\displaystyle{ -\frac{1}{3} }[/math], so by adding the spins of two up quarks and one down quark, the charge of a proton can be derived! The same applies for neutrons, which have one up quark and two down quarks.
Mesons
Mesons are characterized by having an even number of quarks. Even more interestingly, mesons always contain the same number of quarks and antiquarks, which have the opposite (negative) spin of their partner quark. As a result, mesons always have integer spin. Examples of mesons include kaons (K mesons), pions (pi mesons), and eta mesons.
Grand Unified Theory
A grand unified theory (GUT) refers to any theory that can accurately describe the electromagnetic, strong, and weak force in terms of a single force. A theory that accomplishes this would work only at high energies, which may seem inconvenient but does mean that it would help us study the early universe (the grand unification epoch, to be precise). At this point in time, spanning from [math]\displaystyle{ 10^{-43} }[/math] to [math]\displaystyle{ 10^{-35} }[/math] seconds after the Big Bang, all three of the aforementioned forces acted as one force (often referred to as the electronuclear force), before eventually splitting off.
Unified Field Theory
A unified field theory (UFT) is a specific type of GUT that is characterized by using fields to describe fundamental particles and interactions. A field applies a quantity to every position in space, where the quantity is the strength of the field at that point. Most familiarly, a magnetic field is one where a magnetic field strength is spread throughout the universe. Near a magnet, the strength of the field is stronger. Wherever the field strength is stronger, an interaction is more likely to happen. This is demonstrated by putting two magnets close to each other. Once they are close enough, they will attract to each other. On a quantum scale, electrons repel via the electromagnetic field, which is a combination of the electric and magnetic fields. Similarly, a field can be defined for fundamental physical quantities, and a theory that describes how these fields interact is called a field theory. To develop a UFT, you would have to create a GUT that combines the forces using a single field, in the same way that the electromagnetic field combines the electric and magnetic fields.
In the case of a field, the fundamental particles would be represented in a similar way as with wave-particle duality. Instead of discrete particles as you would picture them, the field would be present at every point in the universe, and there would instead be excitations, i.e. "waves" or "ripples", in the field. These excitations would be the fundamental particles like the electron. In a regular field theory, you would have a field for each particle and force, so the force fields would allow for interactions between particles. However, in the case of a UFT, you would instead have a single field that holds the particles and forces.
Theory of Everything
Once gravity has been combined with the other three forces, physicists would have a "theory of everything", one that describes all of the normal matter in the universe. On top of a GUT, it would also have to describe gravity, possibly through a particle known as the "graviton". There have been many attempts to identify this particle, known as the graviton, among which string theory is the most popular candidate for a GUT which would complete the current Standard Model.
It is worth noting once again, however, that our understanding of the universe would still be very incomplete even with a theory of everything, as it would provide no explanation for many scientific phenomena. Among these, the most popular is the question of non-baryonic matter, primarily the dark matter and dark energy which make up 95% of the universe, in addition to various other particles and objects which we don't fully understand yet.
This non-baryonic matter would explain many other cosmological phenomena that could not occur with only the baryonic matter. For example, gravitational lensing studies of the Bullet Cluster, a system of two colliding galaxy clusters, have shown that there is a large amount of dark matter that is invisible to the eye but certainly present, affecting the mass distribution of the system.
Scoring
All portions of the event are assigned point values based on difficulty, with certain questions being designated as tiebreaker problems. Points are awarded for correct answers, and partial credit may be available depending on the question.